The Lanthanides
Overview
The lanthanides (which, along with the actinides, are sometimes referred to as rare earth metals) is a special group of metallic elements, usually shown in the periodic table as the first row in a separate block, underneath the transition metals. Together with the actinides, these elements are known as the f-block elements because most (though not all) have at least one electron in the (n-2)f orbital.
The lanthanides and actinides are sometimes known as the inner transition metals because, like the d-block elements, they have an inner electron shell that is in the process of transitioning between two stable electronic configurations. The f-block elements achieve this by filling the (n-2)f subshell to transition between a stable configuration of eighteen electrons and one of thirty-two electrons.
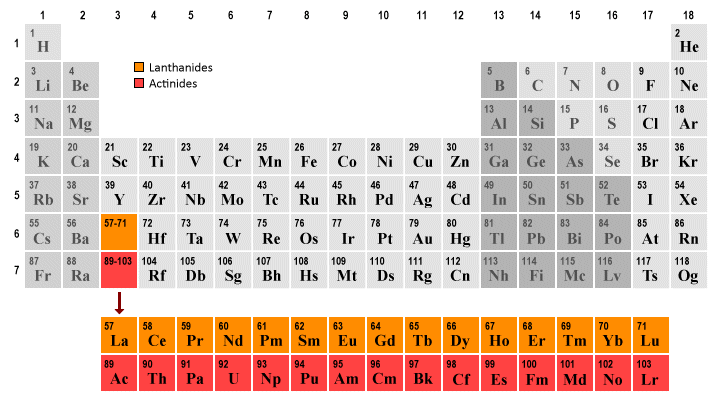
The lanthanides and actinides
Nevertheless, the elements of these groups are quite different from one another in terms of their physical properties and the way in which they interact with other elements. We will look be looking here specifically at the characteristics of the lanthanide series of elements.
Characteristics of lanthanides
The lanthanides consist of the fifteen elements of period six having atomic numbers 57 through 71, starting with the element lanthanum (La) after which they are named. Lanthanum itself is sometimes considered to be a d-block element, because it has no electrons in an f orbital, but it does have one electron in a d orbital. It has also been argued that, because the word lanthanide literally means "like lanthanum", lanthanum itself cannot be a lanthanide.
Lutetium (Lu) is also sometimes considered to be a d-block element, because it has a complete 4f subshell and a single valence electron in the 5d subshell. Nevertheless, both lanthanum and lutetium are usually included in any general discussion of the lanthanides. The table below lists the lanthanide elements and some of their important properties.
| Element | Atomic number |
Symbol | Oxidation states |
Noble gas configuration |
Melting point |
|---|---|---|---|---|---|
| Lanthanum | 57 | La | +1, +2, +3 | [Xe] 5d 1 6s 2 | 920 °C |
| Cerium | 58 | Ce | +1, +2, +3, +4 | [Xe] 4f 1 5d 1 6s 2 | 795 °C |
| Praseodymium | 59 | Pr | +2, +3, +4, +5 | [Xe] 4f 3 6s 2 | 935 °C |
| Neodymium | 60 | Nd | +2, +3, +4 | [Xe] 4f 4 6s 2 | 1024 °C |
| Promethium | 61 | Pm | +2, +3 | [Xe] 4f 5 6s 2 | 1042 °C |
| Samarium | 62 | Sm | +1, +2, +3, +4 | [Xe] 4f 6 6s 2 | 1072 °C |
| Europium | 63 | Eu | +1, +2, +3 | [Xe] 4f 7 6s 2 | 826 °C |
| Gadolinium | 64 | Gd | +1, +2, +3 | [Xe] 4f 7 5d 1 6s 2 | 1312 °C |
| Terbium | 65 | Tb | +1, +2, +3, +4 | [Xe] 4f 9 6s 2 | 1356 °C |
| Dysprosium | 66 | Dy | +1, +2, +3, +4 | [Xe] 4f 10 6s 2 | 1407 °C |
| Holmium | 67 | Ho | +1, +2, +3 | [Xe] 4f 11 6s 2 | 1461 °C |
| Erbium | 68 | Er | +1, +2, +3 | [Xe] 4f 12 6s 2 | 1529 °C |
| Thulium | 69 | Tm | +2, +3 | [Xe] 4f 13 6s 2 | 1545 °C |
| Ytterbium | 70 | Yb | +1, +2, +3 | [Xe] 4f 14 6s 2 | 824 °C |
| Lutetium | 71 | Lu | +1, +2, +3 | [Xe] 4f 14 5d 1 6s 2 | 1652 °C |
As we saw above, the lanthanides are sometimes referred to as rare earths. The term lanthanide itself is thought to come from the Greek word lanthanein, which means "to lie hidden". Despite this, the lanthanide elements exist in greater quantities than many more familiar elements. Cerium (Ce) is the 26th most abundant element in the Earth's crust; neodymium (Nd) is more abundant than gold; and thulium (Tm), the rarest of all the lanthanides, is more abundant than iodine, arsenic or mercury.
The alleged rarity of the lanthanides stems from the fact that extracting them from the mineral ores in which they are found is a lengthy and difficult process. Prior to the Second World War, most lanthanides were extracted from mineral deposits mined in Brazil and India. Today, most of the world's lanthanides are extracted from the huge deposits of bastnäsite - a mineral containing significant amounts of several important lanthanides - found in the Bayan Obo mining district of Inner Mongolia, an autonomous region of the People's Republic of China.
Although all lanthanide-bearing mineral ores contain all of the lanthanide elements, the concentrations vary significantly. The quantities found generally obey the Oddo-Harkins rule, which states that elements with an even atomic number are more common than those with an odd atomic number (the reasons for this phenomenon are beyond the scope of this page and will be discussed elsewhere).
The lanthanides are generally quite soft - some can even be cut with a knife - although hardness increases with atomic number. They have a shiny silvery appearance, but surface oxidation usually occurs quickly when they are exposed to air (the exceptions are gadolinium and lutetium, which only oxidise after prolonged exposure).
Most lanthanides will burn readily in air if the temperature is high enough (around 150 °C) to form oxides. Some, like cerium, will even ignite spontaneously. Lanthanides will also react with water to form oxides, producing hydrogen gas in the process (the reaction occurs more quickly in hot water than in cold water). For this reason, lanthanides are often stored in mineral oil.
Lanthanides tend to form ionic compounds with other elements, typically with highly electronegative elements like oxygen (O) and fluorine (F). We have already noted the ease with which most lanthanides form oxides when exposed to air, or when they come into contact with water.
Interestingly, all of the lanthanides have a similar range of oxidation states, as we can see from the above table. The chemistry of the lanthanides is dominated, in fact, by the +3 oxidation state, in which the two 6s electrons and (usually) one 4f electron are lost to form tripositive (+3) ions. Generally speaking, this is the most stable oxidation state for the lanthanide elements.
Note that for lanthanum, which has no electrons in f orbitals, the +3 oxidation state arises when the two 6s electrons and the single 5d electron are lost. Similarly, both cerium (Ce) and gadolinium (Gd) lose two 6s electrons and a single 5d electron to acieve the tripositive oxidation state.
Less stable +2 and +4 ions may also occur. Cerium, in addition to losing its 6s and 5d electrons, can lose its single 4f electron to form a Ce 4+ ion. Europium (Eu), also usually trivalent, can often form divalent compounds, because the half-filled 4f subshell is relatively stable. Europium is thus less inclined to give up one of its 4f electrons.
Electron configuration
The electron configuration of the f-block elements is interesting because there are parallels to be drawn here with electron configuration of the d-block elements - primarily the fact that the elements in both blocks are transitioning between two stable electronic configurations with respect to the filling of an inner electron shell. The noble gas configuration for the lanthanide elements, from lanthanum to lutetium, takes the following general form:
[Xe] 4f [0-14] 5d [0-1] 6s 2
According to the Madelung rule, the (n-2)f subshell in f-block elements is filled last, i.e. after the (n-1)p and ns subshells have been filled (if you are not familiar with the Madelung rule, see the page entitled "Electron Shells and Orbitals"). A diagrammatic representation of the Madelung rule, showing its predictions for the order in which the orbitals will be filled, is reproduced below.

A diagrammatic representation of the Madelung rule
The Madelung rule is relatively successful in predicting the final electron configuration of an element, but in terms of predicting the order in which the electron subshells actually fill, we can consider it to be somewhat misleading. You should of course make up your own mind concerning this point, but we suggest that, if you have not already done so, you read the page entitled "Transition Metals" in this section, where we explain the reasoning behind this opinion.
The electronic configuration of the f-block elements certainly raises some interesting questions, such as why the 5s, 5p and 6s subshells are filled in preference to the 4f subshell (we will, for the remainder of this discussion, ignore questions related to the precise order in which the subshells fill for individual f-block elements).
A detailed explanation is perhaps beyond the scope of this page, but we'll try and provide a starting point from which which those who are sufficiently interested can undertake further study. Let's start with some basic assumptions (we won't call them "facts" for reasons that will hopefully become apparent):
- Orbitals are filled in order of increasing energy.
- Principal energy levels get closer together as distance from the nucleus increases.
- A subshell in one electron shell may have a higher energy level than a subshell in another electron shell with a higher principal quantum number.
The Madelung rule tells us that the 5d subshell fills after the 4f subshell, so it seems perfectly reasonable that the lone 5d electron is involved in the chemical reactions that occur between lanthanum, cerium, gadolinium and lutetium and other elements. According to the principles outlined above, this electron must have more energy than the electrons in the 4f subshell and be further from the nucleus, so it will be more easily lost.
We also know from experimental data that the two 6s electrons are also lost during ionisation. According to Aufbau principle, this would seem to imply that 6s electrons are both both more energetic and further from the nucleus than electrons in the 4f subshell. And yet, according to the Madelung rule, the 6s subshell fills before the 4f subshell, which would imply the exact opposite.
So, how can the 6s subshell be filled first if it has more energy than the 4f subshell? The truth of the matter is that things are not quite as straightforward as we would like them to be, and there are a number of conflicting theories as to why this apparent contradiction arises.
Shielding and penetration
What does seem clear is that the relationship between the various subshells, in terms of their energy levels, will change as atomic number increases across the lanthanide series. Two of the factors frequently discussed in this context are penetration and shielding. Without going into too much detail, this is all about the attractive force exerted on the negatively charged electrons orbiting an atom by the positively charged protons in the nucleus.
Penetration essentially describes how close (on average) an electron is to the nucleus, and is related to the orbital (the three-dimensional region in which an electron spends most of its time) to which the electron belongs. For example, a 2s electron has greater penetration than a 2p electron, but less penetration than a 1s electron.
Shielding refers to the fact that, not only are electrons further from the nucleus experiencing a weaker force of attraction (simply because they are further away), but they are also repelled by the negative charge of those electrons that are closer to the nucleus, offsetting still further the effects of the nuclear charge.
To summarise, electrons with a high degree of penetration spend most of their time close to the positively charged nucleus, and feel a strong electrostatic attraction. Electrons that are further away from the nucleus not only feel a weaker electrostatic attraction due to their remoteness, but are shielded from the nuclear charge by electrons closer to the nucleus.
The quantum number sum
The aufbau principle tells us that orbitals with the lowest energy levels fill first, and the Madelung rule tells us - or at least claims to tell us - the order in which the various subshells fill. The Madelung rule is based on a simple formula for determining the relative energy level of a subshell. This formula is known as the quantum number sum, and is simply the sum of the principal and secondary quantum numbers (n + ℓ).
The primary quantum number n is the electron shell number. The secondary quantum number ℓ gives us the orbital (s=1, p=2, d=3 and f=4). If two subshells have the same quantum number sum value, the one with the highest value of n will have the higher energy level. The table below shows the quantum number sum for all of the orbitals up to and including n = 7, for all of the known elements.
| Electron shell | Subshell | n + ℓ |
|---|---|---|
| 1 | 0 (s) | 1 |
| 2 | 0 (s) | 2 |
| 2 | 1 (p) | 3 |
| 3 | 0 (s) | 3 |
| 3 | 1 (p) | 4 |
| 3 | 2 (d) | 5 |
| 4 | 0 (s) | 4 |
| 4 | 1 (p) | 5 |
| 4 | 2 (d) | 6 |
| 4 | 3 (f) | 7 |
| 5 | 0 (s) | 5 |
| 5 | 1 (p) | 6 |
| 5 | 2 (d) | 7 |
| 5 | 3 (f) | 8 |
| 6 | 0 (s) | 6 |
| 6 | 1 (p) | 7 |
| 6 | 2 (d) | 8 |
| 7 | 0 (s) | 7 |
| 7 | 1 (p) | 8 |
If we list the orbitals in order of their quantum number sums, we get the following:
1s, 2s, 2p, 3s, 3p, 4s, 3d, 4p, 5s, 4d, 5p, 6s, 4f, 5d, 6p, 7s, 5f, 6d, 7p
Here is a graphical representation of the difference in energy levels of the various electron subshells (note that the diagram is not to scale - differences in energy levels have been exaggerated for the sake of clarity):
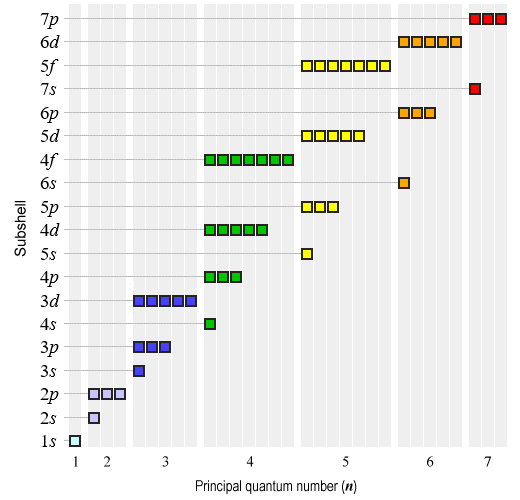
Energy levels in electron subshells
Looking at this sequence, we might expect the lanthanides, most of which have a partially filled 4f subshell, to lose electrons from their 4f subshells before they lose electrons from the 6s subshell. It turns out that in the +3 oxidation state (the predominant oxidation state for the lanthanides), the 6s electrons are lost first. A 4f electron is only lost when there is no 5d electron present (which makes sense, since the 5d subshell is at a higher energy level than the 4f subshell).
The role of f orbitals
The apparent reluctance of 4f electrons to participate in chemical bonding becomes easier to understand when we realise that, from a statistical probability point of view, electrons in 4f orbitals will (most of the time) be closer to the nucleus than electrons from from the 5d and 6s orbitals. The graphic below shows electron distribution probability curves for gadolinium (Gd). The curves represent the electron density, plotted against radial distance from the nucleus in atomic units, for the 4f, 5s, 5p, 5d and 6s subshells.

Electron probabilities P2(r) for the 4f, 5s, 5p, 5d and 6s subshells in Gadolinium (Gd)
Image: Encyclopaedia Britannica Inc.
Even though the 4f subshell has a higher energy level than the 6s subshell, its electrons have greater penetration; most of the time they are closer to the nucleus and thus subject to greater forces of electrostatic attraction. To compound matters, the electrons of the 5s and 5p subshells would appear to be further from the nucleus than the electrons of the 4f subshell, which effectively implies that these atoms take no part in shielding the 4f electrons from the electrostatic force exerted by the nucleus.
It is tempting to ask, in light of the above, why the electrons of f orbitals are involved in ionisation at all, since the electrons of the 5s and 5p subshells would appear to be found further from the nucleus. As you might have guessed, however, when it comes to electron confgurations, things are never quite as straightforward as they might at first seem.
Remember that the 5s and 5p subshells form part of the xenon core, which by definition has a highly stable electron configuration. Part of the reason for this is that electrons from the 5s and 5p subshells often penetrate far more deeply into the xenon core than the electrons of the f orbitals, and spend time very close to the nucleus.
To cut a long story short, it is easier to remove an electron from a 4f orbital than to remove one from a 5s orbital or 5p orbital. Which brings us to the next question. If an electron can be removed from a 4f orbital - apparently with relative ease - why is it that we never see more than one electron from the 4f subshell involved in stable oxidation states?
In a lanthanide atom, the electrons in the 4f orbitals are slightly further from the nucleus than the electrons in the 4s, 4p and 4d orbitals. However, each electron lost to oxidation increases the effective nuclear charge (the degree to which the nucleus attracts electrons), and this increase in effective nuclear charge is, for reasons related to orbital geometry, most keenly felt by the electrons of the 4f subshell.
The loss of a single electron from the 4f subshell - typically during the formation of a trivalent ion - forces a further contraction of the 4f distribution, leaving the remaining 4f electrons closer to the nucleus than those of the 4s, 4p and 4d orbitals. As a result, these electrons are even more tightly bound to the nucleus. Their penetration has increased, their shielding has been reduced, and they are unavailable for chemical bonding.
The lanthanide contraction
The atomic radius of an element is a measure of the size of its atoms, and is usually given in picometres (pm). Whilst the radius of an atom could reasonably be defined as being the distance from the centre of the nucleus of the atom to the outermost boundary of the electron cloud surrounding the atom, this distance is neither well-defined nor easy to measure. Frequently used alternative (non-equivalent) definitions include:
- covalent radius - half the distance between the nuclei of two covalently bonded atoms
- ionic radius - half the distance between two neighbouring ions in a crystal lattice
- metallic radius - the nominal radius of atoms when joined to other atoms by metallic bonds
- van der Walls radius - the radius of an imaginary sphere representing the distance of closest approach for another atom
With the exception of the noble gases, the atomic radius of atoms in a given period decreases as we move from left to right across the table. Each time the atomic number increases by one, an electron is added to the outer electron shell. Because the primary quantum number n remains the same throughout the series, however, each new electron is added to the same electron shell.
For each electron added to the outer shell, a proton is added to the nucleus, increasing the positive nuclear charge and exerting a stronger pull on the electron cloud surrounding the nucleus. The outermost electrons are therefore drawn slightly closer to the nucleus with each proton added, and atomic radius decreases.
The elements in the lanthanide series experience the same pattern of decreasing atomic radius as atomic number increases, but the effect is far more pronounced. The reason for this is that the electrons of the f orbitals, despite spending much of their time deep within the xenon core, do not significantly contribute to shielding.
This lack of shielding allows the nucleus to exert a stronger electrostatic attraction on the 6s electrons in the atom's outer shell, drawing them closer to the nucleus and decreasing the atomic radius to a greater degree than would otherwise be the case - an effect known as the lanthnide contraction. The table below lists the covalent and metallic radii of the lanthanide series of elements.
| Element | Symbol | Atomic number |
Covalent radius (pm) |
Metallic radius (pm) |
|---|---|---|---|---|
| Lanthanum | La | 57 | 207±8 | 187.0 |
| Cerium | Ce | 58 | 204±9 | 181.8 |
| Praseodymium | Pr | 59 | 203±7 | 182.4 |
| Neodymium | Nd | 60 | 201±6 | 181.4 |
| Promethium | Pm | 61 | 199 | 183.4 |
| Samarium | Sm | 62 | 198±8 | 180.4 |
| Europium | Eu | 63 | 198±6 | 180.4 |
| Gadolinium | Gd | 64 | 196±6 | 180.4 |
| Terbium | Tb | 65 | 194±5 | 177.3 |
| Dysprosium | Dy | 66 | 192±7 | 178.1 |
| Holmium | Ho | 67 | 192±7 | 176.2 |
| Erbium | Er | 68 | 189±6 | 176.1 |
| Thulium | Tm | 69 | 190±10 | 175.9 |
| Ytterbium | Yb | 70 | 187±8 | 176.0 |
| Lutetium | Lu | 71 | 187±8 | 173.8 |
The values shown were extracted from periodic table data provided by http://www.ptable.com. Please note that our research has shown that the values given by different sources can vary significantly. This data is only intended to illustrate the general trend for atomic radii to decrease across the lanthanide series.
Applications
Although pure forms of the lanthanide elements have few applications outside of a laboratory setting, there are numerous applications for one or more lanthanides in combination with other elements. Currently, approximately sixteen thousand metric tonnes of lanthanides are consumed annually. Large amounts of various lanthanide compounds, including oxides, are used each year as catalysts in various industrial processes (catalysts are substances that speed up or facilitate chemical reactions while taking no part in the reaction itself).

Lanthanide oxides find numerous industrial and scientific applications.
Clockwise from top centre: praseodymium, cerium, lanthanum, neodymium, samarium, gadolinium
Image: Peggy Greb, US department of agriculture
Other applications of lanthanides include the following:
- The oxides of neodymium, praseodymium and erbium are used as colouring agents in glazes used for the manufacture of ceramic products, producing uniquely coloured transparent coatings.
- Lanthanides are used in combination with other metals to create ferromagnetic alloys for the manufacture of miniaturised components used in all kinds of electronic devices.
- Lanthanides are used to create high-performance alloys with the additional strength, hardness and stability typically required for the structural materials used in many constructon and engineering projects.
- Lanthanides provide the active ions in the luminescent materials used in many optoelectronic devices, such as lasers and optical amplifiers used in fibre-optic transmission systems.
- Cerium oxide in powdered form is used to polish glass.
- Other lanthanide compounds can be added to glass to change its optical properties. Lanthanum oxide is used in camera and binocular lenses to filter out ultra violet and infra red light; praseodymium and neodymium oxides are used in television and computer screens to reduce glare.
- Halides of lanthanide metals such as cerium, dysprosium and holmium are used in conjunction with other metal halides to create high intensity discharge (HID) lamps of the kind used in sports stadiums, street lighting, and television studios.

