| Author: | |
| Website: | |
| Page title: | |
| URL: | |
| Published: | |
| Last revised: | |
| Accessed: |
Amount of substance is one of the seven base quantities defined by the International System of Units. It is in fact the most recent addition to the list, having been added following the 14 th General Conference on Weights and Measures, which took place in 1971. The internationally agreed base unit for amount of substance is the mole (unit symbol: mol). The name mole is believed to have originated in 1894, when the Russian-German chemist Friedrich Wilhelm Ostwald (1853-1932) used the name Mol - an abbreviation the German word Molekül (which means molecule) - for a unit of substance. This later found its way into the English language as mole.
The word substance can have several meanings, depending on the context in which it is used. Before talking about how substance is measured, therefore, it might be useful to define exactly what we mean when we talk about substance in the context of physics - or in the broader context of science generally. For the purposes of the following discussion, we will use the word substance to describe matter that has a defined composition and specific properties.
Essentially, a substance is any pure element or any pure compound. Copper is a pure metallic element (it consists solely of copper atoms) and is therefore a substance. Sulphur dioxide is a pure chemical compound (each sulphur-dioxide molecule consists of exactly one atom of sulphur bonded to exactly two atoms of oxygen). Sulphur-dioxide is therefore also a substance. Salt water - a solution of salt in water - is not a substance. It is a mixture of two substances - salt (sodium chloride), and water. The composition of a salt water solution is not fixed, since the ratio of salt to water may vary considerably.
Atoms, molecules, ions and other particles or groups of particles - collectively known as elementary entities - are the building blocks of matter. Even small amounts of substance are made up of almost unimaginably large numbers of these elementary entities. They are so small that we can't see them, even under a microscope. Obviously, they are not the kind of things we can easily measure or count. There are other characteristics of matter, however, that we can measure, and put a number on. Things like mass, volume, temperature, and pressure, for example. The mole provides us with a convenient way to relate these quantities to a specific number of elementary entities.
If we know the mass of a sample, we can calculate how many moles of substance it represents. With this information, we can determine how many elementary entities it contains with a reasonable degree of accuracy. This is important in pretty much all branches of science, but it is especially important in chemistry. If we know, for example, that two atoms of element A will react with one atom of element B to form compound C, we can measure out two moles of element A and one mole of element B to ensure that the reaction will produce the maximum amount of compound C. To put it another way, moles help us to maximise the conversion efficiency of a chemical process.
Essentially, the mole gives us a handy way of counting out a large number of things, whether they are atoms, molecules, electrons, or jelly beans. It's a bit like a "dozen" in that sense. As you are no doubt aware, a dozen means twelve of something. For example, we can have a dozen eggs, a dozen rabbits, or a dozen amphibious landing craft. If we have a mole of something, it means that we have 6.022 × 10 23 of whatever that something is. That's a very large number. If you write it out in full, it looks like this:
602,200,000,000,000,000,000,000
Actually we have rounded the value off to three significant figures to keep things tidy, but hopefully you get the idea. A mole of jelly beans would occupy the same space as approximately two trillion Empire State buildings - give or take the odd Empire state building! That says something about how big a number it is - and how small atoms must be, if there are that many of them in twelve grams of carbon - that's roughly the same as the mass of two heaped teaspoons of sugar.
The amount of substance we are dealing with in a particular situation can be defined as the number of elementary entities present. An elementary entity can be an atom, a molecule, an ion, an electron, or some other particle or specified group of such particles. You can think of an elementary entity as a the smallest amount of a substance that can exist and yet still be identified as belonging to that substance - for example, a single copper atom, or a single molecule of sulphur dioxide.
Knowing exactly what kind of elementary entities we are dealing with in a given situation is very important from a stoichiometric point of view. Stoichiometry (from the Greek stoikhein meaning element, and metron, meaning measure) is the part of the science of chemistry that deals with calculating the relative quantities of chemical substances that will be involved in a particular chemical reaction (a literal translation would be the measure of elements).
It is very important to understand that, when we talk about an amount of substance, we are taking about some number of elementary entities. It is also very important to understand that, when we talk about an amount of substance, we must specify exactly what elementary entity is involved. This is necessary in order to avoid ambiguity. For example, consider oxygen. Oxygen is an element, so we might assume that the elementary entity for some amount of oxygen would be the oxygen atom. At standard temperature and pressure, however, two oxygen atoms combine to form molecular oxygen (O2 ), a diatomic gas (also known as dioxygen).

Oxygen exists naturally as a diatomic molecule
There are in fact seven diatomic elements, most of which you have probably heard. They are hydrogen (H2 ), Nitrogen (N2 ), Oxygen (O2 ), Fluorine (F2 ), Chlorine (Cl2 ), Iodine (I2 ), and Bromine (Br2 ). Ambiguity can be avoided in cases like this by replacing the term substance with the name of the entity, or by stating the formula for the substance (or both). For example:
In a chemical compound like methane, the bonds between the atoms are called covalent bonds. This essentially means that the atoms bond with one another by sharing electrons, because it gives them more stability. Hydrogen atoms have one electron, but need one additional electron to become stable. Carbon atoms have six electrons, but need four additional electrons to become stable.
A methane molecule is created when four hydrogen atoms bond with one carbon atom. Each of the four electrons belonging to the hydrogen atoms is paired with one of the four electrons belonging to the carbon atom. This effectively means that each hydrogen atom gains one electron, while the carbon atom gains four electrons. The end result is that each atom gets the additional electron(s) it needs to achieve stability, and everybody is happy.
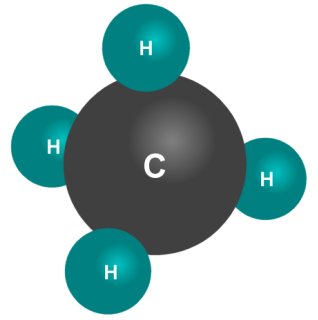
A methane molecule has one carbon atom and four hydrogen atoms
Oxygen, chlorine and methane are all examples of substances that can exist in nature as discrete molecules. The covalent bonds that form between the atoms of a discrete molecule are strong, but the intermolecular forces - the forces of attraction and repulsion between neighbouring molecules - are relatively weak. As a consequence, substances consisting of discrete molecules tend to have low melting/boiling points. Not all chemical compounds, however, consist of discrete molecules.
As well as covalent bonds, some molecules have another type of bond called an ionic bond. Ionic bonding almost always occurs between a metal and a non-metal. In an ionic bond, electrons are transferred completely from one atom to another, generating two oppositely charged ions. The metal loses electrons to become a positively charged ion called a cation, while the non-metal gains those electrons to become a negatively charged ion called an anion. The cations and anions thus formed are bonded to each other by electrostatic attraction.
One chemical compound with which you will certainly be familiar is sodium chloride (NaCl) - better known as common salt. Sodium chloride is an ionic compound, because the bonds between the sodium and chlorine atoms are ionic. Sodium chloride does not exist as discrete molecules. In solid form, the ions form a lattice structure in which each ion is surrounded by six ions of the opposite charge, as illustrated below. In an aqueous solution (i.e. when dissolved in water), both the sodium ions (Na+) and the chloride ions (Cl-) are surrounded by water molecules.
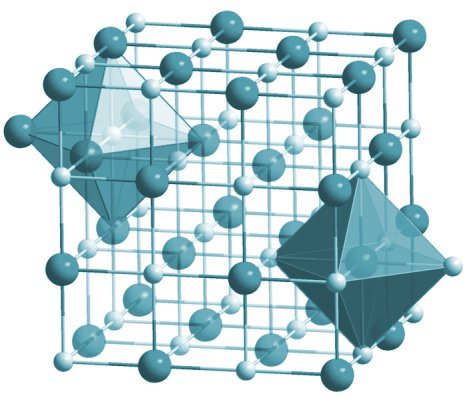
The crystalline structure of sodium chloride
How, then do we specify an "elementary entity" for sodium chloride? We stated above that an elementary entity can be considered to be the smallest amount of a substance that can exist and yet still be identified as belonging to that substance. When sodium and chlorine react together to become sodium chloride, a single atom of sodium donates an electron to a single atom of chlorine. The ratio of sodium and chloride ions in the resulting crystal lattice (or in solution) is one-to-one. An "elementary entity" of sodium chloride can therefore be considered to consist of one sodium ion and one chloride ion. For this reason, we tend to refer to the elementary entities of ionic compounds like sodium chloride as formula units rather than molecules.
As we have already mentioned, the SI unit for amount of substance - sometimes called chemical amount - is the mole. But what exactly is a mole? Well, we also mentioned that the amount of substance was determined by how many elementary entities of a substance (atoms, molecules, or whatever) we have. In fact, a mole of substance contains a very specific number of elementary entities. That number is defined by something called the Avogadro constant (NA ).
The Avogadro constant was originally defined as being the number of atoms in exactly twelve grams (12.0 g) of carbon-12 (12 C) - the most common isotope of carbon. Each carbon-12 atom consists of six protons, six neutrons and six electrons. The reason for choosing carbon-12 is largely historical (if you want to know more about this topic, much of the relevant history is discussed in the page entitled "The Avogadro Constant" in this section). A decision was taken by the 26th Conference of the BIPM in 2018 to fix the value of the seven fundamental constants on which all units in the International System of Units are now based, including the Avogadro constant which is now defined as follows:
NA = 6.022 140 76 × 10 23 mol −1
The important thing to note here is that the relative atomic mass of an element (which is determined experimentally) is given in terms of a unit of mass known as the unified atomic mass unit (symbol: u). Although this is a non-SI unit, it has been accepted for use with the International System of Units. One unified atomic mass unit is approximately the mass of a single nucleon (nucleons are protons and neutrons - the particles that form the nucleus of an atom). It is defined as one-twelfth of the mass of an unbound neutral atom of carbon-12.
Why is this important? Well, we know that a mole of any substance has N elementary entities. For argument's sake, let's assume we want to know the mass of one mole of copper. A mole of copper will, by definition, contain N copper atoms. If we knew the mass of one atom of copper, we could simply multiply this value by the Avogadro constant (NA ) to get the mass of one mole of copper. The mass of an atom, known as its atomic mass, is measured in daltons. One dalton is equivalent to one-twelfth of the relative atomic mass of a single carbon-12 atom. The International System of Units defines this value as 1.660 539 040 (20) × 10-27 kg.
We need to be a little bit careful here, because the atomic mass of a copper atom will vary significantly from one isotope to another. For the purposes of this exercise, well use the relative atomic mass for copper, which is the average atomic mass of all the isotopes of copper, weighted in respect of the abundance of each isotope on Earth. The relative atomic mass for copper is 63.546 daltons. Our calculation will thus be as follows:
63.546 Da × 1.660 539 040 × 10-27 kg × 6.022 × 10 23 mol -1 = 0.063545 kg = 63.545 g
Note that we have limited the precision of the values used in our calculation in order to keep things simple. Our answer will therefore be subject to a slight rounding error, but it is accurate enough for our purposes. Note also that the weight of one mole of copper in grams is almost identical to its relative atomic weight in daltons. This is no coincidence. Now let's look at the relevant section of the periodic table:
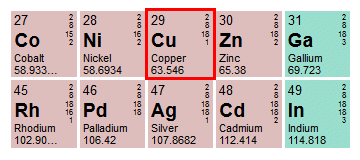
Copper has a relative atomic mass of 63.546 Da
We have highlighted the entry for copper (Cu). For every entry in the periodic table, the relative atomic mass of the element (in daltons) is located underneath the name of the element. As you can see, the value given for copper is 63.546, which is no surprise because we already knew the value. What should occur to you now, however, is that we don't need to do any calculations in order to find the mass of one mole of an element like copper. All we need to do is consult the periodic table and obtain the relative atomic mass for that element. This number will give us the mass of one mole of the element in grams!
Before we proceed, we should point out that the term standard atomic weight is sometimes used in periodic table publications instead of relative atomic mass, but they mean the same thing. The definition of relative atomic mass is "the ratio of the average mass of atoms of an element . . . to 1/12 of the mass of an atom of carbon-12 (known as the unified atomic mass unit)". Because there may be several naturally occurring isotopes of a particular element, the average mass referred to here is the weighted average of the mass of all atoms of that element.
We have seen how easy it is to find the mass of a mole of atoms, but supposing we are dealing with diatomic elements such as oxygen or nitrogen? Or a chemical compound such as sulphur dioxide? Actually, it is not as difficult as you might think. Let's deal with the simplest case first - that of the diatomic element. We'll use chlorine gas as an example.
Like oxygen, chlorine is a diatomic gas. At standard temperature and pressure, two chlorine atoms combine to form molecular chlorine (Cl2 ). Unlike oxygen, chlorine gas has a yellow-greenish colour and a strong, distinctive smell. It is a highly reactive gas that can be extremely dangerous to the point of being lethal if a sufficient quantity is inhaled. In fact, it was used as a chemical weapon in the First World War - where it earned the name "mustard gas" - by both sides. Anyway, here is the relevant section of the periodic table, with the entry for chlorine highlighted:
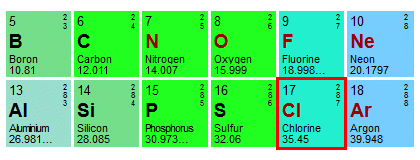
A chlorine atom has a relative atomic mass of 35.45
We can see that the relative atomic mass of a single atom of chlorine is 35.45. Remember, however, that we want to find the mass of a mole of diatomic chlorine. Our elementary entity in this case will be a molecule of chlorine gas (Cl2 ), which consists of two chlorine atoms bonded together to form a molecule. Once we start dealing with substances that are not composed of single atoms, we can't really use the term relative atomic mass any more. Instead, we refer to something called the relative formula mass. The relative formula mass of our chlorine molecule will be the relative atomic mass of two chlorine atoms, which will be 70.90. The mass of a mole of chlorine gas will therefore be 70.90 g.
That was easy, wasn't it? But suppose we are dealing with chemical compounds made up of two or more different kinds of atoms? I'm guessing you may already have some ideas about how that works, but let's look at an example anyway. This time we'll look at methane (CH4 ) - an abundant and naturally occurring hydrocarbon that forms the main component of natural gas. As we saw above, a methane molecule consists of one carbon atom and four hydrogen atoms.
So, how do we calculate the relative formula mass of a methane molecule? If you think we need to add together the relative atomic masses of one atom of carbon and four atoms of hydrogen . . . you would be right! Here are the relevant sections of the periodic table with the entries for hydrogen and carbon highlighted:

We will add together the relative atomic masses of one carbon atom and four hydrogen atoms
From the table we can see that the relative atomic masses for hydrogen and carbon are 1.008 and 12.011 respectively. Our calculation to find the relative formula mass of one molecule of methane (CH4 ) will therefore be as follows:
12.011 + 1.008 × 4 = 16.043
The mass of one mole of methane is thus 16.043 g.
The molar mass of a substance is defined as the mass of that substance divided by the amount of substance (in moles). The SI unit for molar mass is kg/mol (kilograms per mole), although for historical reasons (and because it is often simply more convenient), molar masses are almost always expressed using g/mol (grams per mole). By definition, the molar mass of an elemental substance is its relative atomic mass (a dimensionless quantity) multiplied by the molar mass constant (which is defined as 1 g/mol). In other words, it is just the relative atomic mass of the element in grams per mole (g/mol). For example, the molar mass of copper (Cu) is 63.546 g/mol.
Finding the molar mass of a diatomic substance is simple enough. We know that oxygen gas (O2 ), for example, exists in nature as discrete diatomic molecules. An oxygen molecule is created when two oxygen atoms each share a pair of electrons to form a double covalent bond. The molar mass of O2 is thus just the relative atomic mass of oxygen, multiplied by two, multiplied by the molar mass constant:
15.999 × 2 × 1 g/mol = 31.998 g/mol
Finding the molar mass of a chemical compound is almost as straightforward, except that we need to use something called the relative formula mass (which we mentioned earlier), also sometimes referred to as the relative molecular mass (strictly speaking, the term relative formula mass is the correct term to use, since not all chemical compounds exist as discrete molecules). Suppose we want to find the molar mass of water (H2 O). The relative formula mass of water will be the sum of the relative atomic masses of two hydrogen atoms and one oxygen atom. Hydrogen atoms have a relative atomic mass of 1.008 and oxygen atoms have a relative atomic mass of 15.999, so we get:
15.999 + 2 × 1.008 = 18.015
The molar mass of water is thus 18.015 g/mol. The upper case letter M is sometimes used to denote molar mass, so we could write this very concisely as:
M(H2 O) = 18.015 g/mol
We can also calculate the molar mass for mixtures of chemical substances using mole fractions (also known as amount fractions). A mole fraction is defined as the amount of a constituent substance (in moles) divided by the total amount of all constituent substances in a mixture. The sum of all of the mole fractions in a mixture will be equal to one.
Suppose we want to make up a mixture of two organic compounds. For argument's sake let's say we require a mixture made up of two types of sugar - one part sucrose (C12 H22 O11 ) and three parts glucose (C6 H12 O6 ). This looks tricky, but it's actually fairly straightforward. First of all, we need to find the relative formula mass of each ingredient. For sucrose (the chemical name for the kind of sugar you might have in your tea or coffee), we have:
12.011 × 12 + 1.008 × 22 + 15.999 × 11 = 342.297
And for glucose (the stuff that circulates in your bloodstream as blood sugar), we have:
12.011 × 6 + 1.008 × 12 + 15.999 × 6 = 180.156
The molar mass of our sucrose/glucose mixture is calculated as follows:
0.25 × 342.297 + 0.75 × 180.156 = 220.691 g/mol
In other words, one mole of sucrose/glucose mixture will be made up from the following amounts of each substance:
0.25 M(C12 H22 O11 ) = 0.25 × 342.297 g = 85.574 g
0.75 M(C6 H12 O6 ) = 0.75 × 180.156 g = 135.117 g
One important thing to note here is that our mixture does not consist of one part of sucrose to three parts of glucose by mass. It consists of one part of sucrose to three parts of glucose by amount of substance. In other words, one mole of our mixture will consist of 0.25 × NA elementary elements of sucrose (C12 H22 O11 ) and 0.75 × NA elementary elements of glucose (C6 H12 O6 ).
Calculating the molar mass of a mixture of gases is just as straightforward. The main difference is that quantities of gas are usually given as volumes rather than masses (it's easier to measure the volume of a gas in a laboratory than to weigh it). In fact, the volume ratio of gases in a mixture of gases will be the same as the ratio of the gases in terms of the amount of substance of each gas present in the mix (i.e. the mole fraction). This is because Avogadro's law - named after the Italian physicist Amedeo Carlo Avogadro (1776) - tells us that the volume of a gas at a given temperature and pressure is proportional to the number of molecules present, regardless of the kind of gas involved.
According to Avogadro's law, for example, a given volume of ozone gas will contain exactly the same number of molecules as an identical volume of oxygen gas, despite the fact that an ozone gas molecule contains one more atom than an oxygen gas molecule. Furthermore, the same volume of helium gas will contain exactly the same number of atoms (helium is a monatomic gas).
Just as for mixtures of solid substances, the molar mass of a mixture of gases will depend on both the molar masses of the constituent gases and on the mole fraction each gas represents. If you are at all curious you may, at some time in your life, have wondered about the exact composition of the air that you breathe. As you can probably imagine, the answer will vary somewhat, depending on geographical location, altitude, humidity, and factors such as the level of various pollutants in the air.
The general consensus, however, is that dry air is made up of (approximately) 21% oxygen, 78% nitrogen and 1% argon. There are a number of other gases present in trace amounts, including carbon dioxide, hydrogen, helium, neon, krypton and xenon, but for the purposes of this exercise we will ignore these. Let's suppose we want to find the molar mass of dry air. First, we will find the relative formula mass of each gas (remember that oxygen and nitrogen are diatomic gases). For oxygen (O2 ), we have:
15.999 × 2 = 31.998
For nitrogen (N2 ), we have:
14.007 × 2 = 28.014
Argon (Ar) is a monatomic gas, so we have:
39.948 × 1 = 39.948
The molar mass of dry air is thus calculated as follows:
0.21 × 31.998 + 0.78 × 28.014 + 0.01 × 39.948 = 28.970 g/mol
Find the amount of substance in a sample:
| Amount of substance (mol) = | Mass of sample (g) |
| Molar mass of substance (g/mol) |
The amount of substance in a sample is calculated by dividing the mass of the sample by the molar mass of the substance. For example, suppose we have thirty grams of potassium (K). The molar mass of potassium is 39.0983 g/mol, so the amount of substance in our sample (in moles) is calculated as follows:
| 30 g | = 0.7673 mol |
| 39.0983 g/mol |
Note that for a sample of gas, we are usually given the volume of the sample rather than the mass. Fortunately, Avogadro's law comes to our assistance here. This law states that equal volumes of all gases, at the same temperature and pressure, contain the same number of particles (regardless of the mass of an individual particle, and regardless of whether the particles in question are atoms or molecules).
Avogadro's law also tells us that the volume of one mole of any gas (known as the molar volume) is twenty-two-point-four litres per mole (22.4 l/mol) at standard temperature and pressure (273 K, 1 atmosphere). For gases, our formula will become:
| Amount of substance (mol) = | Volume of sample (l) |
| Molar volume of substance (l/mol) |
Suppose we have just produced two hundred and fifty millilitres (250 mL) of carbon dioxide (CO2 ) at standard temperature and pressure in a laboratory experiment. How many moles of this gas do we have? (Remember that the relative formula mass of carbon dioxide is not relevant to the problem). The molar volume of any gas at standard temperature and pressure is 22.4 l/mol (you might also see it given as 22.4 dm 3/mol), so the amount of substance in our sample (in moles) is calculated as follows:
| 0.25 l | = 0.011 mol |
| 22.4 l/mol |
Find the mass of a sample:
Mass of sample (g) = Amount of substance (mol) × Molar mass of substance (g/mol)
If we know the number of moles of substance in a sample, we can calculate the mass of the sample by multiplying the amount of substance (in moles) by the molar mass of the substance. For example, suppose we have three and a half moles of glucose (C6 H12 O6 ). The molar mass of glucose is 180.156 g/mol, so the mass of our sample (in grams) is calculated as follows:
3.5 mol × 180.156 g/mol = 630.546 g
Find the volume of a sample:
Volume of sample (l) = Amount of substance (mol) × Molar volume of substance (l/mol)
This is usually only likely to be a requirement if we are dealing with a sample of a gas, but it could equally apply to solids or liquids. If we know the number of moles of substance in a sample, we can calculate the volume of the sample by multiplying the amount of substance (in moles) by the molar volume of the substance (in litres per mole). Suppose we have one-eighth of a mole of hydrogen at room temperature and pressure (298 K, 1 atmosphere). The molar volume of any gas at room temperature and pressure is 24.45 l/mol, so the volume of our sample (in litres) is calculated as follows:
0.125 mol × 24.45 l/mol = 3.056 l
Find the number of elementary entities in a sample:
Elementary entities like atoms and molecules are obviously far too small to be counted, but we've been given a pretty good estimate in the shape of the Avogadro constant (NA ) of how many elementary entities there are in a mole of substance. This allows us to calculate the number of elementary elements we are dealing with for a given sample of a substance, providing we are given either the amount of substance in the sample in moles, or the mass of the sample in grams, or the volume of the sample (in the case of a gas) in litres.
This is the formula for when we are given the number of moles of substance in a sample:
Number of elementary entities = Amount of substance (mol) × NA
Let's work out how many atoms there are in three moles of sodium (Na). Remember that the answer will be a pure number - there are no units involved. Here is the calculation:
3 mol × 6.022 × 10 23 mol -1 = 18.066 × 10 23
If we are given the mass of a sample in grams, we need to divide this mass by the molar mass of the substance, and then multiply the result by the Avogadro constant:
| Number of elementary entities = | Mass of sample (g) | × NA |
| Molar mass of substance (g/mol) |
Suppose we want to know the number of molecules in thirty-six grams of sucrose (C12 H22 O11 ). Note that the elementary entity in this case is a fairly complex carbohydrate molecule. In fact, you have already come across this substance - a common form of sugar - in a previous example. We have already calculated the molar mass of sucrose as 342.297 g/mol. Our calculation is thus:
| 36 g | × 6.022 × 10 23 mol -1 = 0.105 × 10 23 |
| 342.297 g/mol |
The procedure to find the number of elementary entities in a sample of gas is similar, except that we will probably be given the volume of the sample in litres. For a sample of gas, the type of gas involved is unimportant (it could even be a mixture of two or more gases). What is important is that we make sure we use the correct molar volume (even though this will be the same regardless of the type of gas, it will vary with temperature and pressure).
For a given volume of a sample in litres, we need to divide this volume by the molar volume of the substance, in litres per mole at the given temperature and pressure, and then multiply the result by the Avogadro constant:
| Number of elementary entities = | Volume of sample (l) | × NA |
| Molar volume of substance (l/mol) |
Let's suppose we want to find the number of carbon dioxide molecules in a sample of gas consisting of five litres of carbon dioxide (CO2 ). For this example, we will assume that the sample is being held at room temperature and pressure (298 K, 1 atmosphere). We have already seen that the molar volume of any gas at room temperature and pressure is 24.45 l/mol. Our calculation is therefore as follows:
| 5 l | × 6.022 × 10 23 mol -1 = 1.231 × 10 23 |
| 24.45 l/mol |
For a temperature and pressure for which the molar volume of a gas is not known, it can be calculated easily enough using the following standard equation (this is a rearranged form of the ideal gas equation):
| Vm = | V | = | RT |
| N | P |
where Vm is the molar volume in litres per mole, V is the volume of the gas in litres, n is the amount of substance in moles, T is the temperature of the gas in kelvins, P is the pressure of the gas in atmospheres, and R is the gas constant, which has a value of 0.082057 L atm mol -1 K -1. The rather convoluted unit associated with the gas constant means litre atmospheres per mole per kelvin. Don't worry if that doesn't mean much to you at the moment. Note, however, that there are several different versions of the gas constant. The correct one to use will depend on the units used for pressure, temperature and volume. This is often a source of confusion for students - and a few teachers!