| Author: | |
| Website: | |
| Page title: | |
| URL: | |
| Published: | |
| Last revised: | |
| Accessed: |
Mass is one of the base quantities defined by the International System of Units, and is a measure of how much matter an object contains. We usually measure the mass of an object by weighing it, although mass and weight are actually two different things. The internationally agreed base unit for mass is the kilogram. Commonly encountered multiples and sub-multiples of mass include the metric tonne (one metric tonne is equal to one thousand kilograms) and the gram (one gram is equal to one thousandth of a kilogram). Note that the adjective metric in front of the word tonne can usually be dropped - it is only there to distinguish the tonne from the ton (note the different spellings), which is the name usually applied in the United Kingdom to a unit of 2,240 pounds (1,016 kilograms).
To further complicate things, a ton in the USA or Canada is defined as 2,000 pounds (907 kilograms), and a metric tonne is called a metric ton in the USA. In an attempt to clarify matters, the British ton (2,240 pounds) is sometimes called a long ton, while the North American ton (2,000 pounds) is sometimes called a short ton. For the purposes of this web page, the word tonne will always refer to a metric tonne (one thousand kilograms).
At some point in your life you have probably weighed yourself on a set of bathroom scales. You might have used kitchen scales to weigh the ingredients for a meal. You have probably also used scales of some kind in science lessons at school. Physicists use similar devices to find the weight (and subsequently the mass) of various things, too. The main difference is that the devices used by physicists are usually more sophisticated, and more accurate, than the kind of equipment you will find in your home or in a school laboratory.

You probably have a set of bathroom scales at home
So, if we are using scales to measure weight, what is the difference between weight and mass? This is a question that confuses a lot of people. Essentially, weight is a measure of the effect of gravity on something. Mass, as we have already mentioned, is a measure of how much matter something contains. To illustrate this, suppose you weigh yourself at home and find that you have a "weight" of seventy-five kilograms. Now suppose that you are suddenly beamed aboard the International Space Station (ISS). You would be virtually weightless, because you would be in orbit around the Earth and, for all practical purposes, no longer subject to the Earth’s gravitational field.
Has your mass changed? No, because your body contains exactly the same amount of matter on board the International Space Station as it does here on Earth. The only thing that has changed is the force exerted on that matter by the Earth's gravitational field. This makes the task of measuring mass a little more complicated than we might at first imagine. How do we measure mass, for example, if we are on the International Space Station - or any other orbiting spacecraft for that matter? Let's just say that it is possible, but it's a topic that will be dealt with elsewhere. For now, we will assume that we are not going to be beamed aboard an orbiting spacecraft any time soon.
Before proceeding further, it might be informative to review exactly how the kilogram - the base unit of mass - is defined. The kilogram was initially defined in 1795 as being the mass of one cubic decimetre of water at the melting point of ice. This meant that mass, as a quantity, was dependent on both the meter (the base unit of length) and on the physical properties of water. It was redefined in 1889 as the mass of the international prototype kilogram (IPK), a solid metal cylinder made from a platinum-iridium alloy (ninety per cent platinum, ten per cent iridium) and having both a height and a diameter of 39.17 millimetres. The edges of the cylinder are chamfered to minimise wear. The cylinder (together with six identical copies of it) is stored in an environmentally controlled safe inside a secure vault at the International Bureau of Weights and Measures, located in Sèvres on the outskirts of Paris, France.
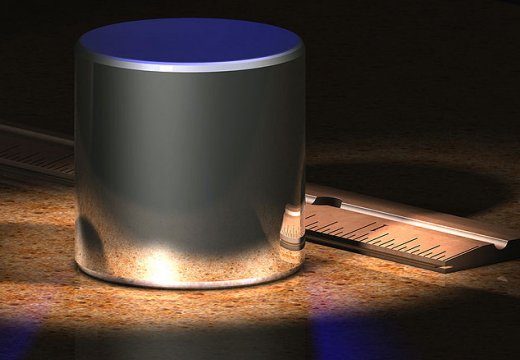
Computer-generated image of the IPK created using Cobalt CAD software by Greg L
The alloy used to make the cylinders was chosen because of its hardness, high density, resistance to oxidation, good electrical and thermal conductivity, and low magnetic susceptibility. Use of the IPK to define the kilogram means that is defined ad hoc, i.e. without reference to any other base unit. There have been several proposals for redefining the kilogram once more, one of which involves defining it in terms of the Planck constant. Official copies of the IPK have been made available to various nations to enable them to establish their own national standards. The original IPK and its official copies are compared roughly every forty years in a periodic verification process, the aim of which is to determine whether any change of mass has occurred in the IPK or any of its copies.
Following the 23rd General Conference on Weights and Measures (CGPM) in 2007, the International Committee for Weights and Measures (CIPM) was tasked with investigating the use of natural constants as the basis for all units of measure, rather than the artefacts that were then in use. Consequently, by 2018 six of the seven base units in the International System of Units had been redefined. The one exception was the kilogram. Following the 26th CGPM, it was agreed that the kilogram would be redefined in terms of three fundamental constants, namely the speed of light c, the Planck constant h, and the resonant frequency of the caesium atom ΔνCs .
Defining the kilogram in terms of these three constants had been a theoretical possibility for many years, since we know courtesy of Albert Einstein, that energy and matter are related. The Planck constant can be used in the definition because it relates the energy in one quantum (photon) of electromagnetic radiation to the frequency of that radiation. Essentially, a photon's energy is equal to its frequency multiplied by the Planck constant. The problem was that, until very recently, the value of the Planck constant could not been determined accurately enough for it to be used to define the kilogram. That has now changed, and the Planck constant has become one of the the seven defining constants used by the International System of Units (SI), with a fixed value of h = 6.626 070 15 × 10−34 J s.
The requirement for the other two fundamental constants becomes clear when we consider that the units of the Planck constant are joule-seconds (J·s). Given that the joule itself is expressed in units of kilograms per square metre per second squared (kg m2 s-2), we can also express the Planck constant in units of kilograms per square metre per second (kg m2 s-1). The metre can be defined in terms of a fundamental constant (the speed of light c), as can the second (the frequency of the caesium 133 atom ΔνCs ). According to the SI Brochure (2019):
"The kilogram, symbol kg, is the SI unit of mass. It is defined by taking the fixed numerical value of the Planck constant h to be 6.626 070 15 × 10−34 when expressed in the unit J s, which is equal to kg m2 s−1, where the metre and the second are defined in terms of c and ΔνCs ."
An earlier proposed redefinition, equivalent to the above, reads like this:
"The kilogram is the mass of a body at rest whose equivalent energy equals the energy of a collection of photons whose frequencies sum to [1.356392489652×1050] hertz."
The kilogram may also be expressed directly in terms of the defining constants:
| 1 kg = | (299 792 458)2 | hΔνCs | ≈ 1.475 521 4 × 1040 | hΔνCs | |
| (6.626 070 15 × 10−34)(9 192 631 770) | c2 | c2 |
The distinction between weight and mass is the source of endless confusion among students new to physics. The confusion is understandable, since we tend to use the terms mass and weight interchangeably in everyday life. The important thing to remember is that the mass of an object does not change, regardless of whether the object is here on Earth, on the Moon, on some other planet, or simply floating around in space. But how do we measure the mass of an object in environments where the gravitational acceleration is very different from that in effect at the Earth's surface?
The standard acceleration due to gravity on the Earth's surface has an approximate value of nine-point-eight-one metres per second per second (9.81 m/s 2), which is factored into the design of kitchen and bathroom scales. If you took a set of bathroom scales to the Moon, you would no longer be able to use them to measure your mass, because the gravitational acceleration on the surface of the moon is only 1.625 m/s 2, which is roughly one sixth that of earth.
It turns out that we cannot avoid this problem entirely even if we stay on our home planet, because gravitational acceleration on the Earth's surface varies significantly, depending on where you are. The value at the equator, for example, is 9.78 m/s 2. At the north or south poles, the value is approximately 9.83 m/s 2. If we just need to measure out the ingredients for a recipe, this variation is not really a problem. When the accuracy of our mass measurements is critical, however, we need some way of eliminating errors due to local variations in gravitational acceleration. The most common solution to this problem is to use an instrument called a balance.
The balance is different from the kind of scales used in a kitchen or bathroom. When you step onto a set of digital bathroom scales, they measure your weight, i.e. the downward force acting on your body due to gravity. The figure displayed by the scales, however, is effectively your mass, i.e. the value of that force divided by the value of Earth's gravitational acceleration. A balance, as the name suggests, works by balancing the weight of an object of unknown mass against the weight of a known mass (a set of test weights, for example). Once the balance reaches equilibrium, the unknown mass can be determined, because it will be equal to that of the known mass.
The advantage of using a balance to measure mass is that any local variation in the acceleration due to gravity will affect both the weight of the known mass and the weight of the object whose mass is being measured to the same degree, whereas a set of scales that relies purely on force measurement must be carefully calibrated to reflect the value of acceleration due to gravity in a particular location. The main disadvantage of a balance is that it does not produce instant results. The value of the known mass needs to be adjusted until its weight equals that of the unknown mass, which can take a certain amount of time.
Although early hunter-gatherer societies had little need for a system of weights and measures, the development of agrarian societies living in permanent settlements changed all that. Goods were bartered, or bought and sold, between individuals and between communities. The value of goods was determined according to quantity. Livestock was often valued on a per-head basis, but fresh produce, and commodities such as rice or grain, and raw materials such as timber and metal ores, were measured in terms of volume or weight.
You may be familiar with terms such as grain and carat in the context of weight measurement. These terms have survived from a time when containers would be filled with seeds, which would then be emptied out in order to determine their volume. The grain is believed to have been the earliest unit of mass, and is based on the weight of a grain of wheat. The carat is another early unit of mass, which was based on the weight of a carob seed, and is still sometimes used today in connection with the weight of pearls and precious stones (you may also have heard the term karat used in connection with the purity of gold alloys).
A balance type scale may have been used originally simply to compare weights, as opposed to determining the actual weight (or mass) of something. There is evidence to suggest that such scales were used during, and probably before, the second millennium BCE. Although no actual scales seem to have survived from this period, stone cubes discovered among the remains of ancient settlements in the Indus river valley (which is in modern day Pakistan) have been found to have weights that are multiples of a common denominator, suggesting that they may have been used as balance weights. Stones of slightly more recent origin, and bearing markings denoting weight, have also been found in Egypt. All the evidence suggests that, up until the seventeenth century CE, most weighing apparatus consisted of some form of balance scale.
In its most basic form, the balance scale consisted of two plates or pans, each suspended from one end of a beam. The beam itself was typically balanced on a central fulcrum. The weight of an object was found by placing it on one of the pans. Weight-setting stones were then placed on the other pan until the beam was level, which meant that the two pans were in equilibrium. The weights of the weight-setting stones were then added together to get the weight of the object being weighed.
The spring balance (or spring scale) is generally considered to have been invented in (circa) 1770 by the English spring maker Richard Salter, and was a major innovation. It is based on Hooke's Law, named after the English scientist Robert Hooke (1635-1703), who formulated it. Hooke's Law states that the force needed to extend a spring is proportional to the distance the spring is extended from its rest position. Thus, the amount by which a spring can be stretched by an object suspended from it will be in direct proportion to the weight of the object.
The spring balance came into widespread usage from about 1840 onwards, when it was adopted by the Royal Mail for weighing letters and packages. Royal Mail employees could work far more quickly with the spring scale than with traditional balance scales, because weight values could be read immediately without the need for adding or removing weights. Although generally less accurate than modern electronic scales and balances, spring scales are still often used in schools and laboratories because of their durability and low cost.
It has been known since at least the early nineteenth century that the electrical resistance of some materials changes when they are deformed by subjecting them to an external force. A material that has such properties can be used to make a device called a load cell. If a constant voltage is applied to a load cell, any change in the force exerted on it will result in a change in the current flowing through it. It is therefore possible to indirectly measure the amount of force being applied to the load cell by measuring the current flowing through it. By the end of the twentieth century, it became possible to manufacture load cells of sufficient quality to be used in the manufacture of digital scales.

A typical digital kitchen scale
Load cells are also used in the manufacture of modern strain gauges. Strain gauges were first invented in the late 1930s by engineers in California carrying out research into the effect of seismic activity on various structures. A strain gauge can be used to measure the amount by which a metal or concrete beam bends when a weight is placed on it. Consequently, they are used in the construction of weighbridges capable of measuring the weight of heavy goods vehicles and railway trucks. Several strain gauges are employed, each measuring the amount of stress the weighbridge is being subjected to at a particular point. These measurements are then added together and translated into the appropriate weight units. Modern weighbridges designed to measure the weight of heavy goods vehicles usually report the weight on each axle of the vehicle.
The spring balance is a device used to measure weight, i.e. the downward force exerted by a mass undergoing acceleration due to gravity. As the name suggests, the main component of a spring balance is a spring. In a traditional style spring balance (see the illustration below), the spring is mounted in a frame. One end of the spring is fixed to the top of the frame. A hook located below the frame is attached to the other end of the spring. A ring or a second hook at the top of the assembly allows it to be hung from a suitable fixed point (typically an overhead beam or metal framework). The item to be weighed is then suspended below the balance using the hook, which causes the spring to be stretched. The amount by which the spring is stretched will depend upon the weight of the item.

An Ohaus Model 8004-MN spring balance
A pointer attached to the spring moves up and down in the centre of the frame as the spring stretches and contracts. Each side of the frame is marked with a graduated scale. Typically, the graduations on one side denote weight (in Newtons or pounds-force), while those on the other side denote mass (in grams or kilograms). When no load is attached to the scale, the spring is at rest and the pointer should align with zero. Once a load is added, the spring will stretch by an amount that is proportional to the applied force, and the pointer will move downwards. The force (i.e. the weight of the load) and the mass corresponding to it can be determined by reading the graduated scale on each side of the frame at the position where the pointer comes to rest.
According to Hooke's law, the force F (in Newtons) exerted on a spring by stretching it is the product of the distance X (in metres) by which the spring is extended beyond its normal length (i.e. the length of the spring when no force is acting upon it) and a constant value k called the spring constant. Hooke's law can be stated algebraically as:
F = kX
The value of the spring constant will depend on the spring. If the spring constant for a particular spring is not known, it can be found by applying a known force F to the spring (i.e. by suspending a known weight from it) and measuring the distance X by which the spring is extended beyond its normal length. We can then simply rearrange the formula for Hooke's law as follows:
| k = | F |
| X |
Although a spring balance is primarily intended for measuring force (i.e. weight), it may be possible to calibrate it to accurately measure mass in a known location (using the local value of gravitational acceleration). Whether measuring mass or weight, however, there are a couple of things that should be noted when using a spring balance. First of all, the spring will have a tendency to "bounce" to a greater or lesser degree, depending on its spring constant (which is essentially a measure of how stiff the spring is). This means that when a load is first added to the spring balance, the pointer will oscillate around its final position for a brief time before coming to rest. The scale should not be read until the pointer has settled down.
The second thing to realise is that the elasticity of the spring can change. It can vary slightly with temperature, for example. Most spring balance manufacturers stipulate that accuracy is only guaranteed within a specified temperature range. More importantly, the spring may be stretched over time due to repeated use, or more quickly if excessive force is applied to it. This will lead to incorrect results being obtained, so the spring balance should be periodically tested using known weights. In some cases, it may be possible to recalibrate the balance.
Although most kitchen scales today are digital, you might have come across the kind of thing illustrated below. This essentially works on the same principle as the spring balance we saw above. The main difference is that a rack and pinion mechanism hidden away inside the body of the scales uses the linear movement caused by the extension of the spring to turn a pointer, which moves around the face of a dial on the front of the apparatus. Evenly spaced graduations around the edge of the dial represent weight increments. When the pointer comes to rest, its position on the dial will indicate the weight of the contents of the pan (remember that in this context, for the reasons already mentioned, weight is synonymous with mass).

Simple kitchen scales based on spring balance principle
The illustration below shows a double-pan balance of a type once used in laboratories by Harvard University to carry out experiments in physics and chemistry. This particular example can measure a mass of up to two kilograms (2 kg). It was manufactured by the Ohaus Scale Corporation of New Jersey, USA. As the name suggests, the balance has two pans. The pointer in the middle indicates whether or not the masses on the pans are equal. Before measuring the mass of an object, the pointer must be set to zero. A sliding weight on the horizontal bar connecting the two pans is used for this purpose.
The object to be measured is placed on one of the pans, and standard masses are placed on the other pan, one at a time. The largest standard mass is added first. If this is too heavy, it is removed, and the next largest mass is added. Standard masses are added to the second pan until the masses on both pans balance (this has been achieved once the pointer is once again pointing to zero). The final step is to add together the standard masses used in order to determine the mass of the object being measured.

An Ohaus Harvard two-pan balance, circa 1970 (www.etsy.com)
A triple-beam balance like the one shown below can be used to accurately measure mass in the laboratory. Like the double-pan balance, it works by comparing an unknown mass to a known mass, and is thus unaffected by local variations in gravitational acceleration. It gets its name from the fact that it has three separate beams. A moveable counterweight (sometimes called a poise) of known mass is mounted on each beam, and can slide along it in either direction. Typically, one of the beams will have a counterweight with a mass of one hundred grams (100 g), another will have a counterweight with a mass of ten grams (10 g), and the third will have a counterweight with a mass of one gram (1 g). A triple-beam balance works by balancing the object whose mass is being measured against the counterweights.
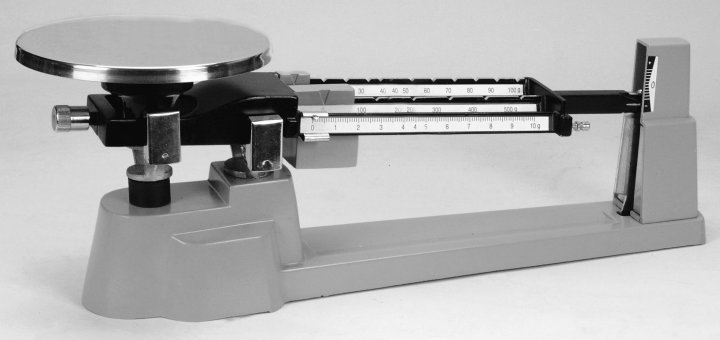
A triple-beam balance
The downward force exerted by each counterweight will depend on the distance between the counterweight and the fulcrum on which the beam assembly pivots - an application of the lever principle first described by the Greek mathematician and physicist (among other things) Archimedes of Syracuse (c.287-c.212 BCE). The further away the counterweight is from the fulcrum, the more downward force it exerts. The balance is calibrated by sliding all three counterweights to the end of the beam nearest the pan. The zeroing screw (usually located underneath the pan) is turned clockwise or anticlockwise until the balance pointer (which is attached to the other end of the beam assembly) lines up with the zero mark, which you can see on the column to the right of the picture. The object to be measured is then placed in the centre of the pan, which will cause the pointer to move upwards, away from the zero mark.
You are now ready to measure the mass of the object. Start by sliding the largest counterweight (i.e. the one hundred gram counterweight) up the beam towards the pointer, one notch at a time. If the pointer drops below the zero mark, slide the counterweight back down the beam by one notch. Let's assume that the object we are measuring has a mass of six hundred and seventy-five grams (675 g). When the counterweight is moved to the seventh notch (the 700 g mark on the beam's graduated scale), the pointer will dip below the zero mark, and you must move the counterweight back one notch (i.e. to the 600 g mark on the scale).
Now move the next largest counterweight (the ten gram counterweight) up the beam towards the pointer, again moving it by one notch at a time. This time, the pointer will not drop below the zero mark until it reaches the eighth notch (the 80 g mark on the beam's graduated scale). Slide the counterweight back down the beam by one notch to the 70 g mark. Complete the process by moving the smallest counterweight (the one gram counterweight) up the beam towards the pointer, until the pointer lines up with the zero mark. There are no notches for the smallest counterweight, so you will have to move it very slowly, and keep checking on the position of the pointer. You should see the pointer line up with the zero mark when the counterweight reaches the 5 g mark. Once the pointer is precisely aligned with the zero mark, the mass of the object is found by reading off the values from the scale on each beam, and adding them together. If we do this, we will get the following:
Mass = 600 g + 70 g + 5 g = 675 g
Although you may not be able to see the scale on the above photograph too clearly, the front-most beam (which is the one on which the one-gram counterweight is mounted) has graduations that go from zero up to ten grams, at intervals of one tenth of a gram (0.1 g). The apparatus is therefore capable of measuring the mass of an object to a precision of at least one tenth of a gram, which is probably good enough for most routine laboratory work. If the quantities of mass to be measured are very small (something weighing only a few milligrams for example), we will obviously need to use a more sensitive instrument.
Before we move on to look at electronic balances, there are a few points that should be mentioned with regard to using balances of any type. First of all, ensure that the balance is level and is placed on a stable surface. Before using the balance, make sure it is set to zero. If measuring the mass of a substance such as a liquid, you will need to put the substance into some kind of container before it can be placed on the balance. Measure the mass of the container first, and then measure the mass of the container with the substance in it. The mass of the container (called the tare) is subtracted from the total mass to give the mass of the substance. Note that with most modern electronic balances, you can place a container on the balance and then zero it. The balance will then measure the mass of the contents only.
Even if it is not strictly necessary to put the object or substance whose mass is being measured into a container, it is considered good practice to use weighing paper when using a balance. In typical usage, a clean piece of weighing paper is placed on the balance pan, and the object or substance to be measured is then placed on the paper. The weighing paper performs two functions. First of all, it prevents contamination due to spillage, and keeps the balance pan clean. Second, because it is made from a special kind of paper that is both moisture resistant and possesses non-stick properties, powdery or grainy materials can be transferred to and from the balance without suffering any loss of mass due to some of it sticking to the weighing paper.
Given a choice between using a container and using weighing paper (for example, you might want to measure the mass of a small quantity of a chemical in powder form), you should use weighing paper. It will be easier to transfer all of the powder off the weighing paper once you have measured its mass, and weighing paper weighs far less than a typical laboratory container, which could be an important factor if your balance has a limited capacity. You will need to measure the mass of the weighing paper separately, and deduct it from the total mass.
There are actually a number of different types of electronic balance, each tailored to a specific need. The kind you are most likely to encounter in a laboratory is the analytical balance, which is used to measure relatively small masses with a high degree of precision. These balances typically have a maximum capacity of a few hundred milligrams, and a precision of between 0.01 and 0.1 milligrams (an electronic balance that can measure extremely small masses - in the order of a few millionths of a gram - is called a microbalance). Because this kind of equipment is extremely sensitive, the accuracy of readings can be adversely affected by environmental factors. Both the design of the balance and the procedures adopted for its use must therefore take such factors into account.
The measuring pan of an analytical balance is housed inside a transparent enclosure in order to prevent the ingress of dust (which can contaminate the sample and adversely affect the operation of the balance), and to ensure that air currents in the laboratory do not affect the readings obtained. Access to the enclosure is typically provided by doors built into the sides and top of the enclosure, which should be closed before weighing commences. The sample to be weighed must be at room temperature in order to ensure that no air currents are generated within the enclosure due to convection.

Kern ACS 80-4 Analytical Balance Scales (www.labanalyticalbalance.com)
Tongs or laboratory tissue should be used when handling sample materials to prevent contamination of the sample. The material to be weighed should be placed in a suitable container (as mentioned above, this should be weighed separately, before the sample is added). If the material is a substance that is likely to absorb moisture from the air, a closed container must be used. The same applies to volatile liquids, to ensure that no mass is lost due to evaporation. Care must also be exercised when weighing ferromagnetic materials, because they can interfere with electronic components inside the balance. Many electronic balances provide a means of suspending samples at a safe distance below the pan, so that they can be weighed without creating electromagnetic interference.
Another very important consideration is whether the balance is being used in an environment where there is a risk of explosion. This is possible wherever there are high concentrations of dust or other fine particles in the air, particularly if they occur within an enclosed space (a coal mine or a grain storage facility, for example). If so, it may be advisable to use a mechanical balance rather than an electronic balance.
The predecessor of the electronic analytical balance was the single pan mechanical substitution balance. In this type of balance, a beam is suspended on a knife-edge, off centre. The long arm of the beam has a counterweight attached to it. The pan is suspended from the short arm of the beam on a second knife edge, together with a number of internal weights that keep the beam at its reference position (i.e. the position it adopts when the weights on both arms balance – sometimes called the null point). When a load is placed on the pan, the internal weights can be removed from (or added to) the short arm one at a time, using external control knobs, until the beam is (approximately) in balance again.
The weight of the load can be found by adding together the values of the internal weights that have been removed from the beam. Any residual difference in weight will result in an angular displacement of the beam from its reference position that is proportional to the difference in weight. The angular displacement is linked to a display that shows the amount of weight that must be added to (or subtracted from) the total weight removed from the beam. Exactly how this is achieved varies, depending on the make and model of the balance.
Electronic analytical balances come in one of two basic forms. The first is known as a hybrid, and is essentially the same as the mechanical balance described above, except that a servo motor is used to restore the beam to its reference point. The amount of electromagnetic force that must be applied in order to achieve this is called the restoration force, and is proportional to the current flowing through the servo motor coil. Like the mechanical balance, the total weight of the load is the sum of the weights removed from the beam, plus the value of the electromagnetic force required to restore the beam to its reference point. In some hybrid balances, the weights are summed electronically and added to the restoration force to get the total weight, which is then displayed as a single output.
Another version of the electronic analytical balance does away with the internal weights altogether. In some cases a beam and a servo motor are still used. The servo motor is coupled to a sensor that can detect any angular displacement in the beam. When a load is added to the balance, the sensor detects the change in the beam’s orientation, and current is supplied to the servo motor in order to restore the beam to its reference point. The amount of current required is proportional to the total amount of electromagnetic force required to balance the load.
The electromagnetic force balance is a more recent development, and does not require a beam or weights. The pan is suspended above a circular electromagnet at its null point, essentially floating above it. Light passing between the electromagnet and the pan is detected by a sensor, which controls the amount of current being fed to the electromagnet. When a load is added to the pan, the pan is displaced downwards towards the electromagnet, and the amount of light reaching the sensor is reduced. The current to the electromagnet is increased until the amount of light reaching the sensor has been restored to its original level. The amount of current supplied to the electromagnet is proportional to the electromagnetic force required to keep the pan at its null point.
It should be noted that, although the kind of device we are describing is called a balance, it works by measuring the force required to counter the weight of the mass being measured, rather than the actual mass itself. The analytical balance must therefore be carefully calibrated in order to compensate for local variations in gravitational acceleration. This is achieved using a known mass, which is either built in to the balance or supplied separately by the manufacturer. Many modern analytical balances are self-calibrating (calibration may occur when the balance is switched on, at predetermined time intervals, or when environmental conditions change, for example).
We have already said that mass and weight are two different things. Weight is the force exerted on an object by a gravitational field. We can think of the weight W of an object as a downwards-acting force vector, the magnitude of which is a scalar (one-dimensional) quantity. The vector in this case has two components, one being the mass m of the object, and the other being the magnitude of the local gravitational acceleration a. In order to find the weight of an object, we can use Newton's second law of motion, which describes the relationship between the mass of an object and the force required to accelerate it. This essentially states that the force F acting on an object is equal to its mass m multiplied by its acceleration a. In algebraic terms, this is expressed as:
F = ma
Since the force we are interested in here is the weight of an object, we can rewrite this as:
W = ma
What we really want to know is the mass m of an object, so we will rearrange this:
| m = | W |
| a |
Now for a trick question. Let's assume that the object whose mass we are trying to find is on the surface of the Earth (rather than in orbit, or on another planet). Let's also assume that we have "weighed" the object in question, using an accurate set of kitchen scales, and the scales have come up with a figure of exactly three kilograms (3 kg). Do we need to divide this figure by the gravitational constant to get the mass of the object? No! Kitchen and bathroom scales for domestic use actually display the mass of an object, not its weight. As we mentioned above weight is actually a measure of force, and would therefore be given in Newtons by our weight formula as follows:
W = ma = 3 kg × 9.81 m/s 2 = 29.43 N
Sometimes, we don't actually need to weigh something to find its mass. If we know the density ρ and volume V of a material, for example, we can calculate its mass m using the following formula:
m = ρV
There are some things for which we cannot use a set of scales or a balance to find the mass. For example, how can we find the mass of a ship, or a planet, or a black hole, or a hydrogen atom . . . ? The short answer is that we can't - not by direct measurement, anyway. We have to find some way of determining the mass of such things indirectly.
Imagine putting something the size of a jumbo jet onto a set of scales. Actually, you might have noticed that we didn't mention jumbo jets when we were giving examples of things that couldn't be weighed on a set of scales. That's because they can be weighed on a set of scales. In fact, several sets of scales are used - one for each set of wheels. When an aircraft maintenance crew needs to weight a large aircraft like a jumbo jet, platform scales are manoeuvred in front of each set of wheels, and the aircraft is towed into position. The total weight of the aircraft is obtained by adding together the weights measured on all of the platforms. So, even a jumbo jet can be weighed! In fact, the weight of a commercial airliner is such a critical safety factor that it must be checked every four years by law.

An aircraft can be weighed using platform scales
Ships are quite easy too, even though we can't just "pop them on the scales". That's because the mass of a ship (including its contents) is equal to the mass of the water it displaces when afloat (this is known as its displacement tonnage). All you need to do is calculate the volume of the part of the ship's hull that is underwater, and multiply that volume by the density of the water. Granted, the maths involved can be a little bit tricky, but it can be done. The only thing we need to keep in mind is that the density of water can vary (seawater has a higher density than freshwater, for example). Every cargo ship has markings on its hull called load lines. The load line that is level with (or closest to) the waterline gives an indication of the current displacement tonnage of the vessel.
So, we have ways and means of finding the mass of some fairly large objects, but what about very small objects? What about atoms, or sub-atomic particles for example? In fact, the mass of an electron, or any other charged particle for that matter, can be found by examining its trajectory in a magnetic field. The magnetic field acts on the charged particle in much the same way that gravity acts on a projectile fired from a gun. The more massive an object is, the harder it will be to accelerate, so its path will be bent less than that of a less massive object. Measuring the trajectory of a particle in a magnetic field doesn't actually give us its mass, just the ratio of charge to mass. We need to measure the charge separately, and then do the necessary maths to get the mass of the particle. But hopefully you get the general idea.
Things can get quite complicated in the sub-atomic realm, though. The theory of special relativity, for example, tells us that subatomic particles moving at very high speeds actually increase in mass. The reason for this is that a lot of energy is required to accelerate an object to near light speeds. The speed of light is a kind of cosmic speed limit that cannot be exceeded. Once an object is moving at almost the speed of light, adding additional energy to it will not make it go any faster. Nevertheless, that energy has to go somewhere. In fact, it is added to the object’s mass.
Looking towards the other end of the scale, how do we find the mass of something really, really big, like a planet - our own planet Earth, for example? Well, it is possible, largely thanks to the work done by the English physicist and mathematician Isaac Newton (1642-1726), and English scientist Henry Cavendish (1731-1810). Newton's Law of Universal Gravitation states that the force of attraction between two objects is proportional to the product of their masses divided by the square of the distance between their centres of mass. Since we know the radius of the Earth, we know the distance between the Earth's centre of mass and the centre of mass of an object at the Earth's surface. We also know what the force of attraction is, because it will be the weight of the object in Newtons. There is however still a slight problem. Here is the algebraic expression of Newton's law:
| F = G | m1 m2 |
| r 2 |
Let's say we want to apply Newton's law to find the mass of the Earth. In the equation, F is the force of attraction between the Earth and some object, i.e. the weight of the object, in Newtons. Weighing the object will probably give us its mass (in kilograms), but we can multiply that by the standard acceleration due to gravity (9.80665 m/s 2) to get the weight in Newtons. Let's say that the masses m1 and m2 are the mass of the Earth (which we don't yet know) and our object (which we do know, because we've weighed it) respectively. We also know the radius of the Earth, represented by r, to be six thousand, three hundred and seventy-one kilometres (6371 km). If m1 (the mass of the Earth) were the only unknown variable, we could simply re-arrange the equation to get m1 on the left hand side.
Unfortunately, we also need to know the value of G, which is the Constant of Proportionality in the Law of Universal Gravitation. Luckily for us, in 1797 Henry Cavendish successfully carried out experiments designed to establish a value for this constant, and determined it to be 6.67 × 10 -11 Newtons between two objects each having a mass of one kilogram, and a distance of one meter apart (this equates to 6.67 × 10 -11 m 3 kg -1 s -2, or six-point-six-seven times ten to the minus eleven meters cubed per kilogram per second squared in SI units, for those interested).
We should probably mention the contributions of at least two other people. How do we know the radius of the Earth, for example? For that, we have to thank the Greek mathematician, geographer and astronomer (among other things) Eratosthenes (c.276-c.195 BCE). To cut a long story short, he managed to calculate the diameter of the Earth reasonably accurately by measuring, and then finding the difference between, the Sun's angle of elevation at noon on the summer solstice in two different locations a known distance apart. The first location was the Egyptian city of Syene (known today as Aswan). The second was the Egyptian city of Alexandria.
In fact, Eratosthenes already knew that the Sun would be directly overhead in Syene at this time of day on the solstice, because it was common knowledge that a person looking down a local well at that precise moment would block the reflection of the Sun in the water. He allegedly paid a man to walk from Alexandria to Syene in order to measure the distance between the two cities. Once he had all the information he needed, he used some basic trigonometry to calculate the circumference of the Earth, and thus derived its radius.
The value of acceleration due to gravity at the Earth's surface was discovered by the Italian astronomer, physicist, engineer, philosopher, and mathematician Galileo Galilei (1564-1642), who allegedly arrived at this figure by dropping heavy objects from the top of the Leaning Tower of Pisa, and measuring the time taken for them to fall to the ground (some historians have expressed doubts about whether or not such an experiment was actually carried out, but it's a good story). So, assuming we have an object with a known mass of (let's say) one kilogram, we should be able to calculate the mass of the Earth (m1 ) quite easily. Rearranging the equation for the Law of Universal Gravitation, we get:
| m1 = | r 2F |
| Gm2 |
Substituting the known values, we get:
| m1 = | 40,589,641 × 10 6 × 9.80665 × 1 | = 5.968 × 10 24 kg |
| 6.67 × 10 -11 × 1 |
We have rounded the result off to four significant figures. If you Google the mass of the Earth, you get a figure of 5.972 × 10 24 kg (i.e. 5,972 × 10 21 tonnes). The slight difference is partly due to rounding errors and partly due to the fact that the Earth is not a perfect sphere, so calculating the exact figure at any given time is a little more challenging. Bear in mind also that the Earth's mass can vary. Some scientists think that the Earth actually loses mass at a rate of about fifty thousand (50,000) tonnes per year, mostly due to escaped hydrogen and helium, and despite gaining some forty thousand (40,000) tonnes per year due to space dust and meteors captured by Earth's gravitational field!
The measurement of mass has a long and convoluted history. Physicists (and those working in other branches of science) regularly use scales and balances to find the mass of samples. It happens in laboratories all over the world, every day. Sometimes, however, scientists need to find some other way of determining mass, and they can be quite ingenious in how they go about it, as we have seen. This is the case with very small things like sub-atomic particles that cannot be seen directly, even with an electron microscope. The same applies to extremely massive things, like super-massive black holes. And don't forget things that are both incredibly big and incredibly far away - galaxies that are so far distant from us that the light takes billions of years to reach Earth, for example.

How do you find the mass of a distant galaxy?